Predicting Filtration Performance of Coalescing Filter
Coalescence filtration is the removal of dispersed droplets from a gas or an immiscible liquid using a fibrous filter. Examples of such applications include the removal of aerosolized oil droplets from engine exhaust or water droplet removal from diesel fuel. The captured droplets coalesce and grow inside the filter, and eventually drain from it under gravity. Simulating such a lengthy experiment using the conventional computational fluid dynamics (CFD) methods, e.g., the popular volume-of-fluid (VOF) method, is computationally expensive. This is because such transient simulations require very small time steps (in the order of microseconds) to remain numerically stable.

In this work, we developed a computationally affordable simulation method that circumvents the above-mentioned problem and is also able to predict the collection efficiency and pressure drop of a coalescing filter as a function of its wetting saturation. Our simulation strategy was to first predict the distribution of wetting fluid in the partially-saturated filter, and then simulate the filtration efficiency of the filter at that saturation level using conventional CFD methods. The mapping of wetting saturation from PMM domain to the CFD domain is shown in the figure below,
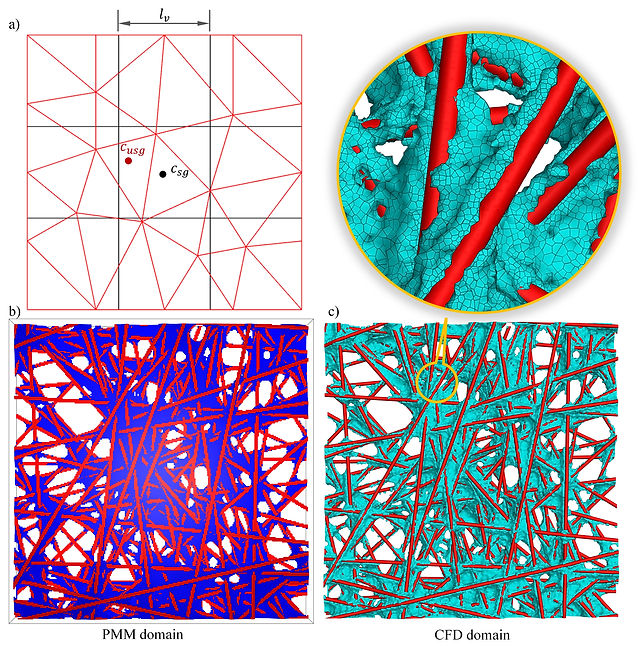
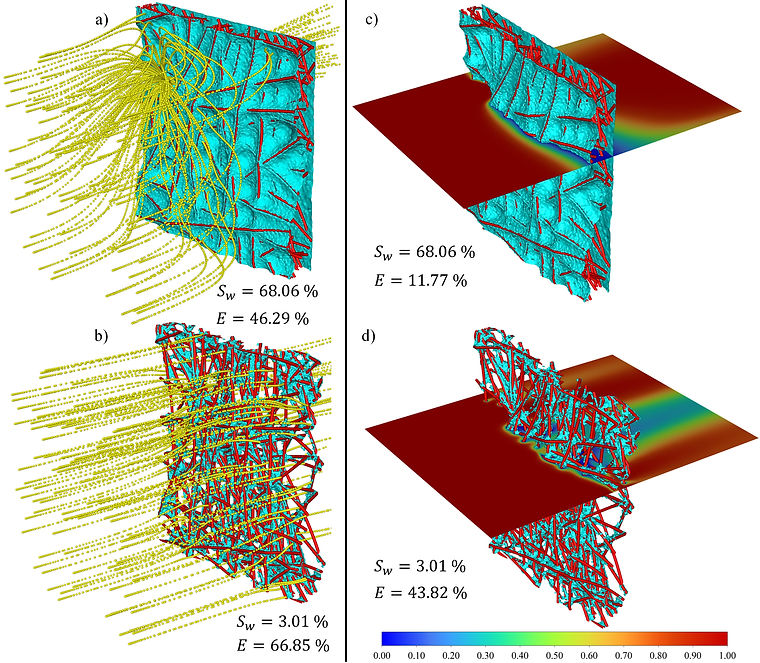
Our simulations revealed an interesting behavior exhibited by the coalescing media: the filter’s collection efficiency is not strongly dependent on media’s wetting saturation. More surprisingly, filter’s collection efficiency can drastically decrease at very high saturations, e.g., 𝑆𝑤 = 68%. This is in contrast to the behavior often observed from aerosol filters loaded with solid particles, where the filter’s collection efficiency increases with particle loading.

The majority of the pores in the high-saturation medium are filled with water, forcing the entire air to accelerate and flow through a single passage. This resulted in particles escaping the filter through this single passage. In contrast, the low saturation medium exhibited a more uniform liquid distribution across the filter area, leading to lower seepage velocities and better interactions between the fibers and the particles, i.e., higher particle capture.
The proposed PMM-DPM approach allows one to predict the collection efficiency and pressure drop of a coalescing filter without needing to commit to time- and resource-intensive multiphase simulations (e.g., VOF). It is worth mentioning that coalescence filtration is a complex multiphase-multiscale problem that is extremely challenging to simulate accurately. Our PMM-DPM approach is novel not because it is very accurate, but because it is a model that is reasonably accurate, and it does not need many months to CPU time on a large computer to provide practical predictions.